日期: 2023 年 9 月 6 日

Encrypting for Apple's Secure Enclave

Encryption, once you have a safe and well-implemented algorithm, is all about the keys. Lose control of your keys, and it’s “Game over, man!” What if we could put our keys somewhere completely out of reach, where even their owner can’t get to them? Yibikeys and HSMs can provide that security, but they’re external devices. However, recent iOS devices and MacBook Pros have something just as good: the Secure Enclave (SE).

Fortunately, using the Secure Enclave for encryption is super well documented. No, wait, it’s hardly documented at all. Trail of Bits encountered this with Tidas, and I recently plunged into the same abyss.

Some demonstration libraries are available on GitHub, but they’re all self-contained. I wanted to generate a message somewhere else, and then decrypt it in the SE. But nothing I found addressed cross-system interoperability.

This led to a long and frustrating detective story. I looked for official docs. Pored over Apple header files and source code. Investigated other example projects, read ECIES papers, and ran down several blind alleys. And of course, I made plenty of stupid mistakes along the way. But I’ll cut to the chase: I eventually figured it out, though it doesn’t quite match the formal specifications. And now, I’ll show exactly how it all works.

Theory

Let me back up a little and explain exactly what I’m trying to achieve. Current MacBook Pros and iOS devices (those with Touch ID and, as I’d forgotten, Face ID) include a Secure Enclave. The Secure Enclave is a separate computer, used for high-security features like TouchID. It comes with its own encrypted firmware, memory, and storage, and hardware-based encryption. Programs talk to the SE through a “mailbox” system, rather than a direct connection. The application places data and commands in a specific memory location, then asks the SE to execute the command. The SE then returns results in the same way.

One feature added in iOS 9, and macOS 10.13, is the ability to store keys and perform cryptography entirely within the Secure Enclave. The application asks the SE to create a public/private keypair. The SE returns the public key (which should then be stored somewhere safe), but it holds onto the private key. Then it can ask “Here, sign this message” and the SE will grab the private key, sign the message, and return the result. Or “Here, decrypt this,” and it’ll decrypt the message using the private key, and return the plaintext. The application itself never has direct access to the private key, so the key should be very secure.

Algorithm Details

Large quantities of data are usually encrypted using a symmetric key. Symmetric algorithms are fast, efficient, and can handled by dedicated hardware. But it uses the same key for encryption and decryption, which means it the Secure Enclave can’t store it. Asymmetric encryption solves this problem, but with a cost: It’s much slower. So in practice, most systems making use of public key encryption use a hybrid approach.

According to Apple’s documentation, the algorithm used for Symmetric Encryption with the Secure Enclave is called:

which refers to an ECIES standard algorithm. The details are a little arcane, but that’s exactly why we’re here. Let’s take this ugly string one part at a time:

  • ECIES: Elliptic Curve Integrated Encryption System - an open standard that defines exactly how to do what we’re about to do

  • Cofactor: Include the elliptic curve’s “cofactor” when completing the Diffie-Hellman key agreement process

  • X963SHA256: Use the ANSI x9.63 key derivation function (KDF), with SHA-256 as an underlying hash function

  • AESGCM: For the final symmetric encryption, use AES in Galois Counter Mode (GCM), a form of authenticated encryption

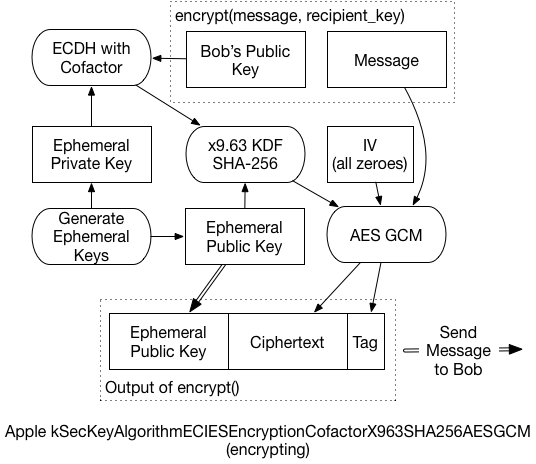
The actual process that takes place is what took some digging to understand. It’s slightly different from the EICES standards, and even from Apple’s published code. Simplified, it works like this:

  • Create a brand-new, “ephemeral” public/private keypair. We’ll use it for only this message and then throw it away.

  • Create a unique shared secret for the message, using the ephemeral private key, and the recipient’s public key. The Elliptic-Curve Diffie-Hellman Key Agreement Process (ECDH) generates the secret.

  • In some circumstances, this shared secret can leak information about the private keys. The x9.63 KDF prevents that, reducing the risk of an attacker decrypting the message. Additionally, the ephemeral public key is used as “Shared Information” for this process.

  • The final key then encrypts the message, and generates an AES-GCM authentication tag.

  • The ephemeral public key, the ciphertext, and the GCM tag are all concatenated, and returned as the final encrypted message.

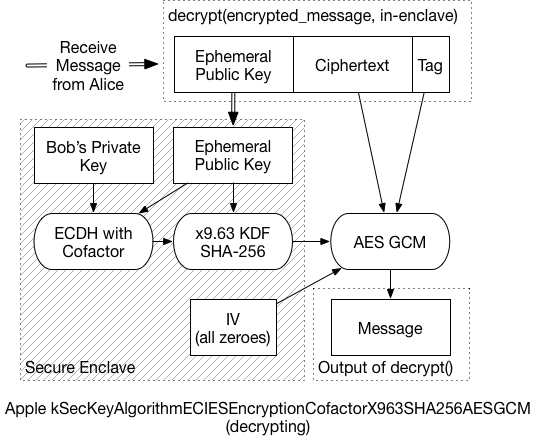
To decrypt the message, the recipient must:

  • Extract the ephemeral public key from the front of the message, and send it to the Secure Enclave.

  • Using the ephemeral key, and the recipient’s private key, the SE performs the same ECDH process. In the end, it should generate the same shared secret as the sender.

  • The SE then applies the x9.63 KDF to generate the symmetric key

  • Using that final symmetric key, the message can be decrypted.

Because the SE communicates in small chunks of data, it could take a while to decrypt a large message. So, that last bit is probably handled by the application processor, and not the SE. That is, the SE would return the final key, and application code decrypts the message. (I didn’t dig enough into this to know for certain, but it seems a reasonable assumption).

As mentioned before, this Implementation var slightly from the expected standard:

  • AES-GCM for encryption instead of XOR

  • Relies on GCM tag instead of separate authentication algorithms

  • Reorders final message to {pubkey} + {ct} + {tag} (instead of key, tag, ct)

Yet, it’s hard to completely fault Apple for straying from the vast array of choices in ECIES. In A Survey of the Elliptic Curve Integrated Encryption Scheme, the authors conclude:

So, it seems unlikely that we’ll ever see any broadly interoperable ECIES implementations. Given that, cherry-picking the best components to create a similar system isn’t much of a stretch. A later paper from the same authors reaches similar conclusions.

Technical Details

The curve generated by the SE in the demo application is a “prime256v1” curve, also known as “SecP256R1”. By reviewing keychain/SecKey.h, I was able to learn some of the deeper technical details. One such detail is the fact that this algorithm I’m spending so much time discussing is now considered “legacy” and shouldn’t be used for new code:

This comment also indicates that the AES-GCM uses the “static public key data” (the recipient’s public key) as additional authentication data (AAD). But it doesn’t work if you include that. So the docs are wrong, or the implementation is broken…or both.

For the preferred “VariableIV” algorithm, the KDF generates the IV, as well as the key. Instead of deriving 16 bytes, the KDF returns 32 bytes: The first 16 are the 128-bit AES key, the following 16 are the IV. For a curve that’s larger than 256 bits, you’d derive 48 bytes – 32 bytes of key material, then 16 bytes of IV. This VariableIV algorithm also does not appear to use AAD.

The KDF itself is actually less of a black box than I’d initially thought. It’s simply a hash of three concatenated values: sha-256( { shared_key } + counter + { shared_info} ):

  • The shared_key is the result of the ECDH function.
  • Shared_info is the ephemeral public key data
  • The counter is a four-byte, big-endian number, starting at ‘0000 0001’
  • The counter increments by one for every block that the KDF produces

Since SHA-256 produces 32-byte outputs, we only need to run through the KDF once. If we have to produce a 2nd block (bytes 33-64), then the counter changes to ‘0000 0002’, and a new hash is generated. And so on.

Example Code

None of this would’ve been possible if I hadn’t found some library to help me with the various primitives. The x9.63 KDF is pretty simple, but I needed to find the ECDH function, and AES-GCM. The last isn’t as widely available in popular python cryptography libraries.

I’m making use of the “hazmat” primitives available in the pyca/cryptography library. I also used PyCryptodome to verify the GCM output. For fun, I replicated the KDF function myself using an off-the-shelf SHA-256 routine (not shown here). So the only black box left (to me, anyway) is the ECDH key agreement phase, but that’s standard too. Plus, the script works with the SE demo code, so I’m confident that everything is working fine.

Again, I’m using this macOS demo application, from GitHub. To use my test script:

  • Start up the demo application, and click on Encryption
  • Then click “Encrypt” to fill the lower box with a ciphertext. We don’t need it, but we do need the public key.
  • Copy the public key displayed in the interface
  • Replace the “bob_pem” value in the script with the public key
  • Run the script, and copy the result into the demo app, overwriting the original ciphertext. (You may need to backspace once to get rid of any trailing newlines).
  • Click “Decrypt”, and if necessary, authenticate via TouchID
  • Gaze in wonder at the properly decrypted message

Here’s the bare minimum of the test script. A full, commented version can be found in this Gist on Github.

上面的例子，介绍了 Python 下编解码 Apple's Secure Enclave 算法的逻辑。那么如何在 Java 下实现相同的逻辑呢？可以参考 iOS-compatible ECIES implementation in Java 中的代码实现(可以点击这里下载代码拷贝)。需要注意的是，工程介绍里面声明实现的是 SecKeyAlgorithmECIESEncryptionCofactorVariableIV* 算法，而我们使用的是  SecKeyAlgorithmECIESEncryptionCofactor* 算法。我们只需要在代码中，加解密的地方传入16位全零的数组，即可兼容两者。

如下：

Changes for Variable IV, Other Curves

I mentioned before that this algorithm has been deprecated by Apple. The recommended algorithm is now “kSecKeyAlgorithmECIESEncryptionCofactorVariableIVX963SHA256AESGCM.” To support this algorithm, which derives a unique IV instead of using all zeroes, we only need minor changes:

This rough demo script isn’t set up to handle curves other than Prime256v1. But since the Secure Enclave only supports 256-bit curves, it’s not really an issue. We’d need to inspect the recipient’s public key, and use the same curve for the ephemeral keys. Then, if the curve is larger than 256 bits, we need to derive a larger symmetric key. In the above snippet, change the length from 32 to 48. The encryption key comes from the first 32 bytes, while the IV is the last 16.

Conclusion

So why is all this cool? Because we can be confident that nobody can read our data without our device. Granted, this presumes that the Secure Enclave is, in fact, Secure. We haven’t yet seen any (public) breaks of SE security, but that doesn’t mean it’s impossible. But since it’s so crucial to iOS and now MacBook security, I expect it’s likely pretty good.

What would be even better is if some decrypted items could remain in the Secure Enclave. Consider a system with many layers of encryption keys. The iOS Data Protection hierarchy is a good example of such a system. If you decrypt a group key with the Secure Enclave, then the key gets returned to the application. An attacker may be able to extract that key from the process' memory space. But if that key never leaves the SE, then there’s no risk of it getting leaked through application memory.

Hopefully features like this are in the works for future versions of iOS and macOS. In the meantime, it’s still a very powerful tool, and definitely worth investigating.

References

参考链接

iOS Keychain: using Secure Enclave-stored keys

One of the great hardware features of iPhone is Secure Enclave — a special hardware element designed to protect user’s sensitive data, including biometric data like fingerprints when device has a Touch ID sensor and face scans in case of Face ID. Secure Enclave ensures that this kind of data is safe even if a hacker gets access to device RAM or disk storage — the thing is that this data never gets to RAM and is never processed by OS or by programs in user space. OS or a program can only interact with Secure Enclave hardware using a predefined set of commands which doesn’t allow access to raw data.

Besides biometric data, Secure Enclave can store cryptographic keys. Such keys are generated inside this hardware element and never “leave” it — there’s no way to get them (unless you break somehow the hardware protection — as for now, there were no reports, suggesting that this is possible).

Currently, only Elliptic-curve cryptography keys can be stored in Secure Enclave. This is an asymmetric cryptography approach so we can talk about public and private keys here. The private key is generated and stored in Secure Enclave. The corresponding public key is available for export and can be transmitted to a communication counterparty or used for encryption locally.

Generating and fetching a key

To generate a Secure Enclave-stored key we can use a SecKeyCreateRandomKey call with special attributes. Here’s how this can be done:

The key type is set to kSecAttrKeyTypeEC and its size is indicated as 256 bits — only this kind of keys can be stored in Secure Enclave presently.

We can additionally use biometry to protect the key. This means that in order to use it, user will be required to go through Touch ID or Face ID authentication (see my previous post about biometry-protected keychain entries). In the fragment of code above we have a requiresBiometry parameter which being set to true enables such protection. This is done by setting the .biometryCurrentSet flag when we create the SecAccessControl instance that we later use to create the key.

Note that at the end of this procedure we’re getting a SecKey instance that we later can use in our program. This, however, doesn't mean that the private key we just created is loaded into RAM. Our SecKey is just a handle object allowing access to the key stored in Secure Enclave.

When we have our key in keychain, we can always load it using a function like this:

We’re getting the key calling the SecItemCopyMatching function and using the same “application tag” value (the kSecAttrApplicationTag field) that we indicated at the key creation stage.

Encryption and decryption algorithm

Now let’s look how we can use the key that we just created. Before we proceed we have to decide what encryption algorithm we’re going to use and check that our current device/OS supports it.

In the official documentation Apple suggests using the following algorithm: eciesEncryptionCofactorX963SHA256AESGCM. This long name basically means that we are usign Elliptic Curve Integrated Encryption Scheme. It refers to the ANSI X9.63 standard. A key derivation function defined in that standard (with SHA-256 hash) is used to generate an encryption key that’s finally used to encrypt the data using the AES-GCM algorithm. The “cofactor” term in the name of the algorithm tells us that some additional measures are taken for protection from certain types of attacks (we won’t delve into details here).

If we take a look at the corresponding constant kSecKeyAlgorithmECIESEncryptionCofactorX963SHA256AESGCMdefined in SecKey.h (see here for example) then we can see that this algorithm is considered “legacy” and the recommended one is kSecKeyAlgorithmECIESEncryptionCofactorVariableIVX963SHA256AESGCMinstead (in Swift it’s eciesEncryptionCofactorVariableIVX963SHA256AESGCM). We’ll use this recommendation in our example. The only difference between these two algorithms is that in the old algorithm an all-zero initialization vector was used to perform AES-GCM encryption. In the newer algorithm the IV is generated together with shared encryption key during the ECDH procedure (see below).

ECIES and ECDH overview

All this sounds a bit scary so let’s first look at the way ECIES works in general. As we’re talking about cryptography, of course we’ll be talking about Alice and Bob. Suppose Bob wants to send an encrypted message to Alice. The following diagram describes how Alice and Bob can in a safe way agree on a shared key that later can be used for encryption by both of them. Here we omit all the technical and mathematical details to make it as simple as possible.

Elliptic-curve Diffie–Hellman (ECDH) key agreement protocol.

Here’s what happens on this diagram:

  • First Bob gets Alice’s public key.
  • Then Bob generates a random value. This random value is also referred as an “ephemeral private key”. “Ephemeral” means that this key will be used only once — for this encryption session only.
  • After that Bob generates a public key for his ephemeral key and sends that to Alice
  • Now Bob generates a symmetrical key that can be used for encryption. For this he uses Alice’s public key and his own ephemeral private key.
  • On the other side Alice can reconstruct the same key using her private key and Bob’s ephemeral public key

So both sides have the same key and Bob can successfully encrypt his message and send it to Alice and she won’t have any problems decrypting it.

This is all nice but how is this related to our case with a key stored in Secure Enclave? We don’t have any network communications in our basic scenario. We just want to encrypt some data. Who is playing the role of Alice and who is playing the role of Bob? We’ll try to answer these questions in the next section.

How does it work in Apple’s crypto-APIs?

Sadly, Apple documentation is quite far from being full and detailed when it comes to this topic. So it takes a bit of guess work and reverse engineering to fully understand what exactly happens when you use these APIs. I can recommend this great post by David Schuetz for detailed description of this matter. It will be especially useful if you’re thinking about some encryption data interoperability (with your backend or other systems).

Here we will focus mostly on the practical side of the problem omiting some low level details. We have already seen how to generate a private key that’s stored in Secure Enclave (makeAndStoreKey method described above). Now let’s look how we can obtain the corresponding public key and how we can check that a given encryption algorithm is supported by the system.

If we have a key returned by the makeAndStoreKey method, then getting the corresponding public key is as easy as calling SecKeyCopyPublicKey and passing the original key as parameter.

Once we have our keys we can check if the algorithm we want to use is supported by the system. Here’s a fragment of code where we get our public key and check that we can encrypt data with it using the eciesEncryptionCofactorVariableIVX963SHA256AESGCM algorithm:

As far as I know it will be supported on all iOS 10+ devices with Secure Enclave, but anyway it won’t hurt you to have such checks in your code.

As you can see SecKeyIsAlgorithmSupported has three parameters: key, operation and algorithm. And all the three parameters are important. You can have different keys, and for each key type different algorithms and operations can be supported. If you experiment a little bit with the code above, you’ll see that if you change the operation to .decrypt, the method will return false. At the same time, if you try it with our private key, then you’ll get true for decryption and false for encryption.

With this information let’s look at our ECIES scheme again. We know that our private key is stored in Secure Enclave, so in our scenario it is Alice who owns (and uses for decryption) the private key, and it is Bob who uses Alice’s public key to encrypt the message.

But what about the ephemeral key? It turns out that it’s generated every time some data is encrypted. And Bob puts the ephemeral public key together with the ciphertext he generates. Then Alice can pick it up, reconstruct the shared key and decrypt the data.

In Apple’s crypto API SecKeyCreateEncryptedData is used to encrypt data and SecKeyCreateDecryptedData is used for decryption. When Bob will call SecKeyCreateEncryptedData, this function will return a CFData object containing the ephemeral public key (generated and used during this particular call) and the actual ciphertext. Now Alice can call SecKeyCreateDecryptedData to decrypt this data. All she needs is her key in Secure Enclave and the ephemeral public key that comes with the data from Bob.

A few more words on the ciphertext generated by SecKeyCreateEncryptedData: since it’s generated by AES-GCM, besides the encypted data itself, it contains an authentication tag (a piece of information used to verify the integrity of the data). At the same time initialization vector (IV) is not put into the output because it’s generated together with the shared key. It means that both parties (Alice and Bob) can reconstruct not only the shared key used for encryption but also the IV used to initialize the AES-GCM procedure (see ECIES/ECDH scheme).

Here’s a short example demonstrating how to call the encryption and decryption functions. For encryption:

And for decryption:

Note that we call SecKeyCreateDecryptedData on a background thread because if our key is biometry-protected, then this call will trigger Touch ID or Face ID authentication UI and won’t return until user authenticates or cancels the authentication. We don’t want to block the main thread while this function waits for user authentication.

Signing and verifying signature

Keys that are stored in Secure Enclave can be used not only for data encryption but also for digital signature. To sign a message you need your private key (stored in Secure Enclave). To verify the signature another party needs the corresponding public key. Of course we suppose that the public key is handed to the other party in a secure manner to avoid man-in-the-middle attack.

For digital signature we will be using the following algorithm: ecdsaSignatureMessageX962SHA256. This means that an ANSI X9.62-compliant version of ECDSA (Elliptic Curve Digital Signature Algorithm) is used and SHA256 is used as a cryptographic hash function. A SHA256 hash value is calculated for the original message and all the other steps of ECDSA are applied to it. By the way, if you already have that SHA256 value calculated, you can use the ecdsaSignatureDigestX962SHA256 algorithm which does exactly the same taking a SHA256 hash value as its input instead of the original message.

This is an example where we show how to calculate digital signature using our Secure Enclave-stored key:

First we check that our digital signature algorithm is supported and then the digital signature value is calculated by the SecKeyCreateSignature function. We call it on a background thread to prevent the main thread from blocking in case our key is protected by biometry.

You can call the sign function presented above in the following ways:

Note that these two calls will give you exactly the same result.

Now, let’s look how we can verify the signature that we just calculated:

Here we also check that the algorithm is supported and then call SecKeyVerifySignature to verify the signatue. This function takes as its arguments the original message and the digital signature that we previously calculated. The function returns true if the signature is valid.

I hope that this article will help you to make your iOS app more secure by applying some of the features coming with secure hardware element that’s included in iOS devices (Secure Enclave).

Full code samples for this article can be found here:

algrid/keychain-sample

If you want to store small bits of generic data in iOS keychain (not EC encryption keys as described here) and protect them with a password or biometry (Touch ID/Face ID), you can take a look at my other posts: Password-protected entries in iOS keychain and Biometry-protected entries in iOS keychain.

参考链接